Integers Lesson 3

INTEGERS - Lesson 3
Good morning students.

You are all very good with computers.

I am sure you have checked the answers from the back of the textbook. You can ask me or check for solutions online.

Textbook to be followed : NCERT

Sources : Youtube

Students you will be able to :

Extend your knowledge of closure ,commutative and associative property to addition and subtraction of whole numbers to integers.

Explanation

CLOSURE PROPERTY :

Closure property under addition –

Integers are closed under addition, i.e. for any two integers a and b, a + b is an integer.

 Example = Explain Closure Property under addition with integers (-8) and 2
Answer = Find the sum of given Integers ;
(-8) + 2 = (-6)
Since (-6) is also an integer we can say that
Integers are closed under addition

Closure property under subtraction –

Integers are closed under subtraction, i.e. for any two integers a and b, a – b is an integer.

Example  = Explain Closure Property under subtraction for integers 10 and 5
Answer = Find the difference of the given integers ;
10 - 5 = 5
Since 5 is also an integer we can say that
Integers are closed under subtraction

COMMUTATIVE PROPERTY

Commuting means interchanging.
Commutative Property explains whether on changing the order of integers in an expression, the result changes or not. 

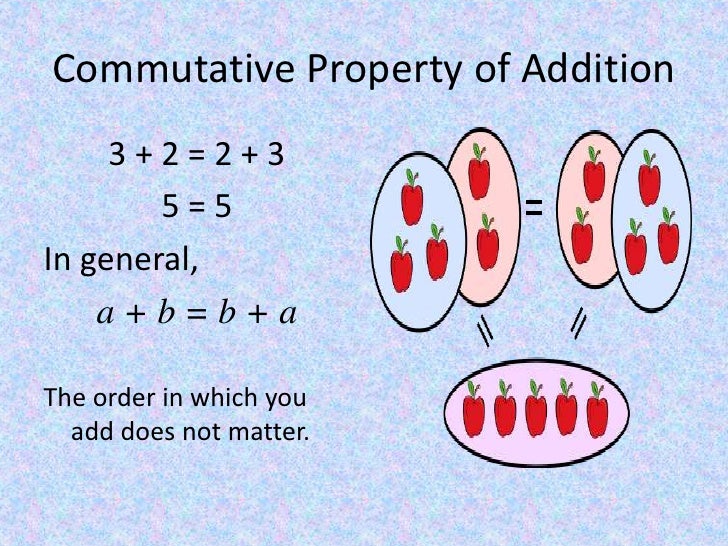
Commutative Property for Addition of Integers 

Let us see if we change the order of integers in an addition expression, the result remains the same or not.  

Example  = Explain Commutative Property for addition of integers (-5) & (-7).
Answer = Given Integers = (-5), (-7) and their two orders are as follows :-
Order 1 = (-5) + (-7) = (-12)
Order 2 = (-7) + (-5) = (-12)
As, in both the orders the result is same i.e (-12),
So, we can say that Addition is Commutative for Integers.

Commutative Property for Subtraction of Integers 

https://i.ytimg.com/vi/Odqi4o_xAsY/maxresdefault.jpg

Let us see if we change the order of integers in subtraction expression, the result remains same or not. 

Example  = Explain Commutative Property for subtraction of integers (-7) & (-17) ?
Answer = Given Integers = (-7), (-17) and their two orders are as follows :-
Order 1 = (-7) - (-17) = 10
Order 2 = (-17) - (-7) = (-10)
As, in both the orders the result is different.

So, we can say that Subtraction is not commutative for integers.

ASSOCIATIVE PROPERTY

Make different groups of whole numbers of a given expression and check if the result of all the groups is the same or not

Associative Property for Addition of Integers

If we make different groups with same given whole numbers, then also the sum in all the groups always remains the same.

Example 1 = Explain Associative Property for addition of 5, 6, 7 ?
Answer = Given Whole Numbers = 5, 6, 7 and their two groups are as follows :-
Group 1 = (5 + 6) + 7
= 11 + 7     = 18
Group 2 = 5 + (6 + 7)
= 13 + 7     = 18
As, in both the groups the sum is same i.e 18
So, we can say that Addition is Associative for integers.

Associative Property for Subtraction of Integers

Example  = Explain Associative Property for subtraction of 5, 6, 7 ?
Answer = 

 Group 1 =    (5 - 6) - 7       = (-1) - 7     = (-8)

Group 2 =     5 - (6 - 7)        = 5 - (-1)     = 6

In both the groups answer is different
So, we can say that Subtraction is not Associative for integers.